Q3 ===================

Q7 ===================
 |
| Unknown Author :-) |

Q15 ===================
Q17 ===================

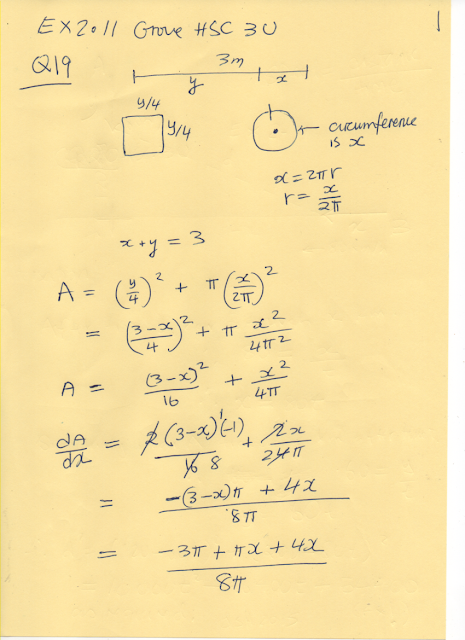
Q19 ===================
The question

Q24 ===================
Q25 ===================
EXTRA EXAMPLE BY JAN HANSEN 2015 ========
MAXIMUM AREA OF A PEN PROBLEM
(c.f. Grove HSC 2U EX 2.9/2.10, Grove HSC 3U EX 2.10/2.11)The farmer's wife, Hazel, got $180$ m of fencing at a sale. Hazel asked her husband, Bob, if he would build a rectangular fence of the largest possible area. Hazel wants to know the dimensions of the rectangle and its area.
Background. Two rectangles can have the same perimeter but have different areas. For example;
The area of the left is much bigger than the right but they both have the same perimeter!
To solve this problem Bob let's the sides of the rectangle be length $x$ and height $y$ as shown.
Then Perimeter $P=2x+2y$. Bob is given the constraint that perimeter $P=180$ m.
Therefore
$ 2x+2y=180$
$\therefore 2y=180-2x $
$\therefore y=90-x $
Area of Pen is $A=xy $
$\therefore A=x(90-x) $
$$\therefore A=90x-x^2 $$
Bob wishes to find the value of $x$ which gives a maximum value for $A$.
'$A$' is a function of $x$.
Bob uses calculus to solve this problem.
Differentiating with respect to $x$ gives (twice),
$${dA\over dx}=90-2x$$
$${d^2A\over dx^2}=-2$$
[REMARK: ${dA\over dx}$ is the same thing as $A'$. In problems involving maximum and minimum values it is more common to use ${dA\over dx}$ to make clear what we are differentiating with respect to. ]
For stationary points: $A'=0$.
$\therefore 90-2x=0$
$x=45$.
When $x=45$, $A=90x-x^2=90(45)-45^2=2025$.
Stationary point is $(45,2025)$.
When $x=45$, ${d^2A\over dx^2}=-2<0$. So concave down.
This means that $x=45$ is a maximum turning point.
Bob tells his wife that the rectangle should be of dimensions $45$ m $\times 45$ m to maximise the area (i.e. a square shape).
The area of the maximum rectangle is $2025$ cm${}^2$.